poltblast.blogg.se - Area of isosceles right triangle with integral

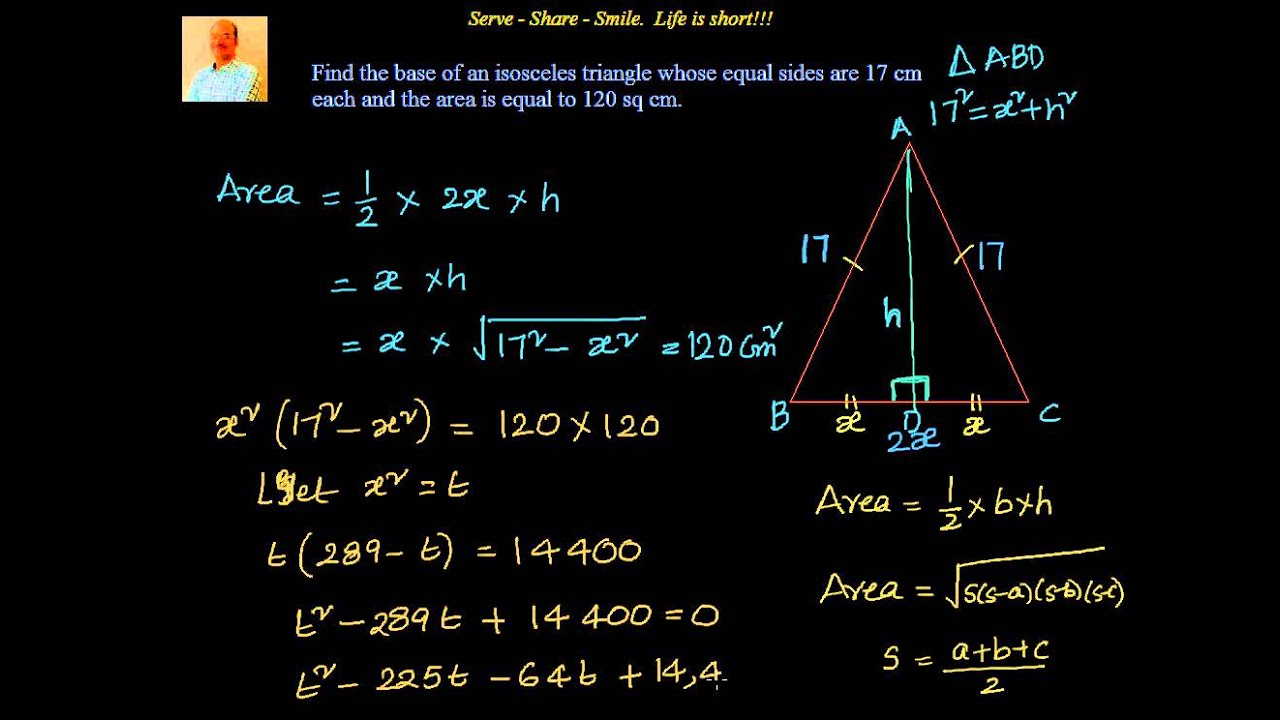
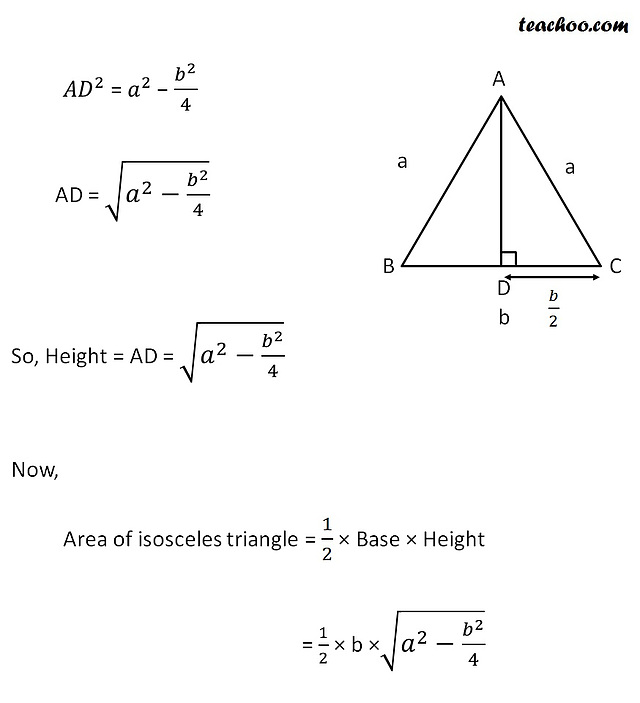
Use trigonometric functions to find the maximum and minimum values of a given function.How can you use trigonometry to calculate the distance between two points on a plane?.How do you convert from radians to degrees and vice versa? Explain the difference between radians and degrees.How can trigonometry help you in your future career?.Use trigonometry to find the area of a triangle.What is the unit circle? How is it used in trigonometry?.How does trigonometry relate to circular motion and harmonic motion?.

What is the law of cosines? How is it used to solve oblique triangles?.

What is the law of sines? How is it used to solve oblique triangles?.

How can trigonometry be used to calculate the height of a building or a mountain?.

Explain why the sine and cosine functions have a period of 2π, while the tangent function has a period of π.

What is the Pythagorean theorem? How is it related to trigonometry?.

Find the missing side of a right triangle if you know one angle and one side using trigonometric ratios.

How do these sides relate to the sine, cosine, and tangent functions?

Draw a right triangle and label the opposite, adjacent, and hypotenuse sides.

Explain the difference between sine, cosine, and tangent.

Here are some examples of journal prompts for Trigonometry: Trigonometry journal prompts can help high school students deepen their understanding of basic concepts, develop their problem-solving skills, and explore real-life applications of trigonometry. It is used in a variety of fields, such as architecture, physics, and engineering. Trigonometry is a branch of mathematics that deals with the study of relationships between the sides and angles of triangles. High school math journal prompts for Trigonometry So if you’re struggling with math, why not give math journal prompts a try? You might be surprised at how much they can help! Over time, this kind of reflection can help you develop a deeper understanding of math and become a more confident problem-solver. Instead of just solving the problems in front of you, you have to engage with the concepts, think about how they connect to other topics, and make connections to real-world situations.

The diameter of the semicircle is determined by a point on the line x + 4 y = 4 and a point on the x‐axis (Figure 2).One reason math journal prompts are so effective is that they require you to think more deeply about the material. The area ( A) of an arbitrary square cross section is A = s 2, whereĮxample 2: Find the volume of the solid whose base is the region bounded by the lines x + 4 y = 4, x = 0, and y = 0, if the cross sections taken perpendicular to the x‐axis are semicircles.īecause the cross sections are semicircles perpendicular to the x‐axis, the area of each cross section should be expressed as a function of x. The length of the side of the square is determined by two points on the circle x 2 + y 2 = 9 (Figure 1). In this case, the volume ( V) of the solid on isĮxample 1: Find the volume of the solid whose base is the region inside the circle x 2 + y 2 = 9 if cross sections taken perpendicular to the y‐axis are squares.īecause the cross sections are squares perpendicular to the y‐axis, the area of each cross section should be expressed as a function of y. If the cross sections are perpendicular to the y‐axis, then their areas will be functions of y, denoted by A(y). The volume ( V) of the solid on the interval is If the cross sections generated are perpendicular to the x‐axis, then their areas will be functions of x, denoted by A(x). You can use the definite integral to find the volume of a solid with specific cross sections on an interval, provided you know a formula for the region determined by each cross section. Volumes of Solids with Known Cross Sections Volumes of Solids with Known Cross Sections.Second Derivative Test for Local Extrema.First Derivative Test for Local Extrema.Differentiation of Exponential and Logarithmic Functions.Differentiation of Inverse Trigonometric Functions.Limits Involving Trigonometric Functions.